The idea of the presence of a ``torrent or flying cloud of charged atoms or
ions'' from sunspots, i.e. matter leaving the
sun and streaming out into space, was first hypothesized by
Fitzgerald [Fit92] and again by Fitzgerald [Fit00] and
Lodge [Lod00].
A refined description of the solar wind was later described by
Chapman and Ferraro [CF31a]
as an idealized model of supersonic
expansion of the solar corona. They also discussed the interaction
of the solar wind with the
earth's magnetic field. Chapman and Ferraro proposed that a current system
would flow on the frontside of the earth's magnetic field. They also
proposed the earth's magnetic field would carve out a ``hollow'' in the
solar stream. This feature was eventually named the magnetosphere by
Gold [Gol59]. Only in the early 1960s was the existence of the solar wind
verified by observations with Russian and American space probes. The name
``solar wind'' and the correct theoretical basis were due to Parker
[Par63].
Measurements of the
positive ions by plasma probes indicated that the flux of particles with
energies exceeding 25 eV is between 
and 
particles 
, with an equal number of electrons present for
electrical
neutrality. At the distance of the earth's orbit around the sun, namely 1 AU
(or 
), the
speed of
the solar wind is usually between 200 and 800 
; the flow
is highly supersonic. The solar wind
carries with it a weak magnetic field amounting to a few nanoTeslas; this is
called the
Interplanetary Magnetic Field (IMF), which was discovered in 1958 with the
Pioneer I satellite as described by Sonett et al. [SSS60].
It is oriented in a direction nearly parallel to the
ecliptic plane but at an angle of approximately 
to a line from the
sun to the
observer at 1 AU. The plasma of the solar wind with an imbedded IMF,
is frequently analyzed using magnetohydrodynamis (MHD).
More simply, the field is
said to be ``frozen in'' to the plasma because the electrical conductivity of
the plasma is very large, so that relative motion between plasma and the
magnetic field becomes virtually impossible. This can be shown in a short
proof using Maxwell's equations, Ohm's law and some vector identities
[Hes68].
The equations of Maxwell
and Ohm's law
can be solved for 
and combined to yield:
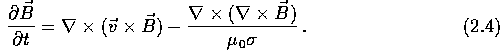
Using the vector identity
and Maxwell's equation
we get
If the plasma is at rest ( 
) Equation 2.7
is reduced to
a diffusion equation with diffusion coefficient 
.
If the conductor occupies a space characterized by length L, the time it takes
for a magnetic field to enter and leave it, is approximately
. For times smaller than 
the field and the
plasma can be considered to move together; this is the case for
solar-terrestrial parameters.
On the other hand, for times very much shorter compared to
Equation 2.7 becomes:
We consider the rate of change of magnetic flux 
through
a moving contour which is given by:
Using the Curl Theorem, the second term can be converted into a surface integral:
which can be combined with the first term to yield
Since Equation 2.8 holds in this case we must have
which means that magnetic field lines are frozen in the plasma and move with it.
The solar wind has a major influence on the ionosphere through
interactions of both the plasma and the IMF with the earth's magnetic field.
The monitoring of solar wind parameters is a vital part of ionospheric
experiments - especially since
the solar wind has time-dependent disturbances associated with it. One such
important disturbance is a coronal mass ejection (CME), in
which massive
amounts of solar plasma jet out from the corona at higher-than-average
speeds and densities [GHM 
74].
Gosling et al. [GBMP90] showed that fast CMEs, carrying
plasma moving faster than the solar wind in front, produce shocks at the
leading edge of the CME and these shocks are associated with large
geomagnetic storms. Another solar wind disturbance in which the IMF varies
slowly and regularly with time over many hours is called a magnetic cloud
[BSMS81, Bur88].
In general, the plasma and IMF conditions in the solar wind
should always be considered when
analyzing ionospheric events; this will be done for the times of the events
in this thesis. [Har92, KR95]
